4.1. Oxygen generation and transfer. The volumetric O2 transfer coefficient.

Opposite to what we find in cultures of bacteria or yeast, in which oxygen supply is a necessity, microalgal culture systems accumulate O2 due to the photosynthetic activity. Oxygen must be evacuated or it will hinder photosynthesis because, as we explained earlier, RUBISCO is an oxygenase as wellas a carboxylase, and would turn ribulose 1,5 biphosphate into glycolate instead of our beloved phosphoglycerate.

Thus, we need to withdraw the generated oxygen at a rate that is high enough to keep the oxygen concentration at bay in the culture broth. Desorbing enough oxygen mans having a degasser which is large enough to keep up with the photosynthetic production. We can also increase stirring at the cost of spending more energy.

The volumetric oxygen transfer coefficient is the key concept here. Some of you may already be familiar with this concept. As we are dealing mostly with liquid, we will refer preferably to the volumetric oxygen transfer for the liquid side, uaually noted KLa.

1. •Correlations: predicting KLa.
   

There is a huge body of knowledge in KLa determination. Since this coeffcient vaties with many culture variables, the a common form for researchers to publicize the result is the use os empirical equations called correlations. The most simple kind of correlations are of the form:

Where Pg/V is the power input per volume unit (W m-3) and vs the superficial gas velocity (m3 s-1 m-2). This tipe of correlation canno take importat factors such as temperature or viscosity. The dimensional analysis can be used to develop better equations, particularly obtaining the Sherwood number as a functiom of the Reynolds and Schmidt numbers.

Questions:

1. • Make a list of physical properties that can influence the KLa.
   

2. • What does the “a” in KLa represent?
   

3. •How does temperature influence KLa?. Does temperature increase the driving force for oxygen desorption?
   

LITERATURE CITED

García-Ochoa F. and Gómez E. (2009) Bioreactor scale-up and oxygen transfer rate in microbial processes: An overview. Biotechnology Advances 27 (2009) 153–176

Transfer rate, driving force and the KLa.

This number represents the proportionality between the rate of oxigen transfer and the driving force. In this case the transfer takes place from the liquid culture to the aeration stream which is usually air. If we call FO2 the oxygen transfer rate per volume unit (eg. in mole·m-3·s-1), from the definion of KLa, we have:

Where CO2 is the oxygen concentration in the culture while the number with the asterisk is the oxygen concentration in the culture corresponding to the equilibriom with the gas phase. Thus we make the transfer take place until the equilibium is attained. This equilibrium concentration is given by the Henry Law.

Which has been written specifically for oxygen but is good for many other gasses. Remember that PO2 is the partial pressure of the gas (oxygen). For oxygen:

This is, at 25ºC (298 K) H=7.916·104 (Pa m3 mole-1). On the other hand, for air xO2=0.21 (mole mole-1). Thus, at PT= 101300 Pa we  have that PO2=21279 Pa. From this, the equilibrium concentration of oxygen at 25ºC and 1 atm is:

Make a note because we will use that many times. Remember that you can calculate the value corresponding to any other gas temperature, pressure or gas composition.

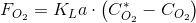
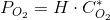
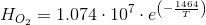
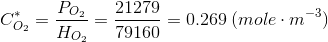
Measuring KLa.

Thre transfer rate is only a part of the picture. To be able to deal with oxygen we need also a mass balance. A way of measuring KLa is to use a device that holds a given amount of liquid phase. If we sparge this device with an oxygen-free gas stream, we can write the following diferential mass balance (0=accumulation+input):

Where CO2 is the oxygen concentration in the culture while the number with the asterisk is the oxygen concentration in the culture corresponding to the equilibriom with the gas phase. Thus we make the transfer take place until the equilibium is attained. This equilibrium concentration is given by the Henry Law.

Which has been written specifically for oxygen but is good for many other gasses. Remember that PO2 is the partial pressure of the gas (oxygen). For oxygen:

This is, at 25ºC (298 K) H=7.916·104 (Pa m3 mole-1). On the other hand, for air xO2=0.21 (mole mole-1). Thus, at PT= 101300 Pa we  have that PO2=21279 Pa. From this, the equilibrium concentration of oxygen at 25ºC and 1 atm is:

I will spae you the math but separating variables, integrating and using the initial condition (t=0,CO2=CO20), we have:

This means that if you plot the logaritmic quotient vs t, you should obtain a straight line which slope is KLa. An experiment of that sort is easy to do with just a oxigen electrode and transmitter and a data logger that can be your own self. We will do one of these experiments in class.ther

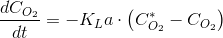
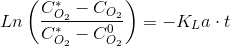
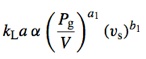
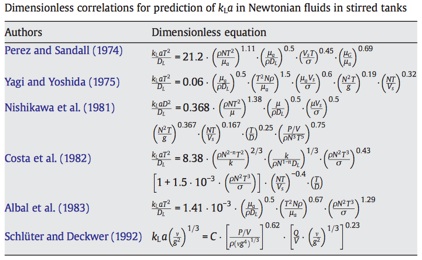
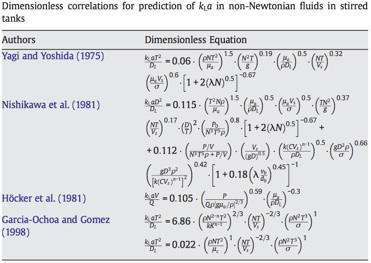
These would be the mass transfer equivalents to heat transfer correlations such as the Dittus-Boelter.

You can find some correlations for mass transfer here. Bear in mind that the tricky thing with KLa and its correlations is that they are quite specific to the systems they have been obtained for.

Sure you are by now wondering, but what on earth is a KLa in microalgal culture systems? Just for your peace of mind, I will let you know that you may be happy with something like 10-20 h-1 (that would be 5·10-3 s-1) but it can vary largely.